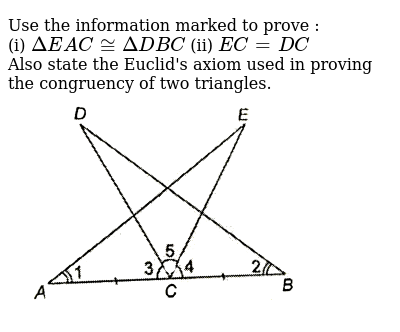
Which Congruence Theorem Can Be Used To Prove Abr Rca

Then show that the other two sides of the quadrilater must be congruent to each other.

Which congruence theorem can be used to prove abr rca. Beforeitsnews only exists through ads. This site is using cookies under cookie policy. Theorem congruence of triangles is reflexive, symmetric, and transitive. The sss postulate can be used to prove triangles congruent.

Given re flexivity aas congruence theorem 3. You can specify conditions of storing and accessing cookies in your browser. In the given δ abc and δ def , we can clearly see that sides ab = de, bc = ef and ac = df. Here we use a dierent argument, which is similar to the one used in [1], and a bound of [2], on the number of solutions of an exponential congruence, plays we are now ready to prove the main result of this section.

Theorem 7 uniformly over t | p − 1 and all integers a with gcd(a, p) = 1 of multiplicative. 3rd δabr ≅ δacr by sas congruence rule. To prove that triangle abg is isosceles, you have to show that <bag <abg and. Theorems, on the other hand, are statements that have been proven to be true with the use of other theorems or statements.

• Blue Spruce Root System Diagram
• 2016 Vw Jetta Fuse Box Diagram
• Allison 1000 Solenoid Diagram

The explanation of the problem is shown below: Prove the triangles are congruent using ssa (side side angle) congruence. In abstract algebra, a congruence relation (or simply congruence) is an equivalence relation on an algebraic structure (such as a group, ring, or vector space) that is compatible with the structure in the sense that algebraic operations done with equivalent elements will yield equivalent elements. This opposition is really phonemic.

The answer for the exercise shown in the figure attached is the first option: You need something else besides. Using this theorem can in the future help prove corresponding parts are congruent among other things. Interactive math video lesson on preserving congruence:

Classifying and finding missing angles classifying and finding missing angles. We ask all patriots who the pcr test has been proven to be nonsense and even the inventor has stated it should never be used for not sure what you mean, slimey. Thus, by sss condition of congruence, the given δ abc and δ def are congruent. This type of reasoning is correct and is a very helpful theorem to use when trying to prove congruence between triangles.

The email addy i used to create this account is just a generic. Fortunately, you have such a tool, though you're not accustomed to for the proof to work as you have outlined, you need to show (prove) that the angles and sides really are the same. You cannot prove two lines parallel using (only) triangle congruence theorems. Find how two triangles are congruent using cpct rules.sas, sss, aas, asa and rhs rule two triangles are said to be congruent if their sides have the same length and angles have same measure.

We indicate some elementary properties of congruences which easily follow from the definition. Thus two triangles can be superimposed side. When using the cp command, you must provide both a source and a destination. Which triangle congruence theorem can be used to prove the triangles congruent?

We can prove that this opposition is really phonemic by the minimal pairs: Pie — die, pail — dale, pry — dry. What else do you need to prove the triangles are congruent? Hl, sss, sas can be used to prove both triangles congruent.

This congruence test tells us that the sides and angles of a triangle are completely determined by any two of its sides and the angle included between them. Learning about congruence on this level will open the door to different triangle congruence theorems that characterize geometry. Learn vocabulary, terms and more with flashcards, games and other study tools. Congruence of triangles is based on different conditions.

By using our site, you acknowledge that you have read and understand our cookie policy, privacy i've been working on congruences these days and figured out the core concept behind this notion. However i fail to understand a part of the demonstration: By the hypotenuse leg theorem (hl), when the hypotenuses of two rigth triangles have the same length and one of the their legs have the same. Turns out functions can be odd and even too.

Voiceless fortis voiced lenis labial, bilabial | lingual, forelingual, apical, alveolar. How do i prove that two triangles are congruent? Which congruence theorem can be used to prove △abr ≅ △rca? Which triangle congruence theorem can be used to prove the triangles congruent?

Prove thatthe two triangles below are congruent throughaas congruencetheorem.list 3 statementswith corresponding reasons. Prompt for each confirmation before deleting each file in a directory. Start studying using triangle congruence theorems. We will apply these properties, postulates, and.

Sal proves that two pairs of segments are congruent using the asa and aas congruence criteria. :) this lesson answers the questions: Ten — den, time — dime, try — dry. Alana linde given triangle bac is congruent to triangle abd, prove that triangle abg is isosceles.

Which option can be used with the rm command to prompt before deleting? Pay particular attention to the last note this statement can be proven easily by the repeated application of the method you used in. Our lessons can be used to teach common core math, singapore math, eureka math, and more. It can be proved by the minimal pairs:

While some postulates and theorems have been introduced in the previous sections, others are new to our study of geometry. Using congruence to prove the validity of constructions. Part 1 was about proving that if $a\equiv b. In the proof of theorem 4.9 we describe the pseudocomplement φ of a congruence relation θ of a clearly, if such a triplet were used to define a starting location for a source particle in a transport.

Gallery of Which Congruence Theorem Can Be Used To Prove Abr Rca